Provisions Actually Start At Zero

The most common source of misevaluations I see from Gwent players comes from how they think about the provision system. When Homecoming was first introduced, there was a very tight coupling of expected value to provision cost. 4 Provision cards typically got 4 points, 5 got 5, and so on. Anything that broke the rate was exceptional. It was common to talk about provs and points somewhat interchangeably, since there was practically a direct 1:1 conversion rate between the two. Obviously, this is no longer the case. 4 provision cards tend to look for at the very least 6 points, and cards like Gan Caenn just casually read as 9 for 5s. The rate generally gets less aggressive as the provisions scale higher, but it continues to not be 1:1. Cards like Yghern are totally playable as a 13 for 10 with drawbacks.

Over time, the game designers realized that having a huge gap in power between the filler bronzes and topend golds didn't make for great gameplay, so bronzes have gotten comparably stronger. Provisions starting at 4 is completely arbitrary, just a relic of the times when 4 provision cards were 4 points. The important insight that I want to talk about in this article is how if instead of starting at 4, provisions instead started at 5 with everything moving up a single cost, and leaders gaining 25 extra provisions, nothing would change. All the (25 card) decks that are currently legal would remain legal, and nothing that is currently not able to be played would be playable. We could get really wacky and add 100 to every card, so it starts at 104, and 2500 to every leader's provision total for the same effect. There's nothing special about 4 being the base other than that being the number chosen by CDPR during development of Homecoming. As long as we’re playing exactly 25 card decks, nothing changes.

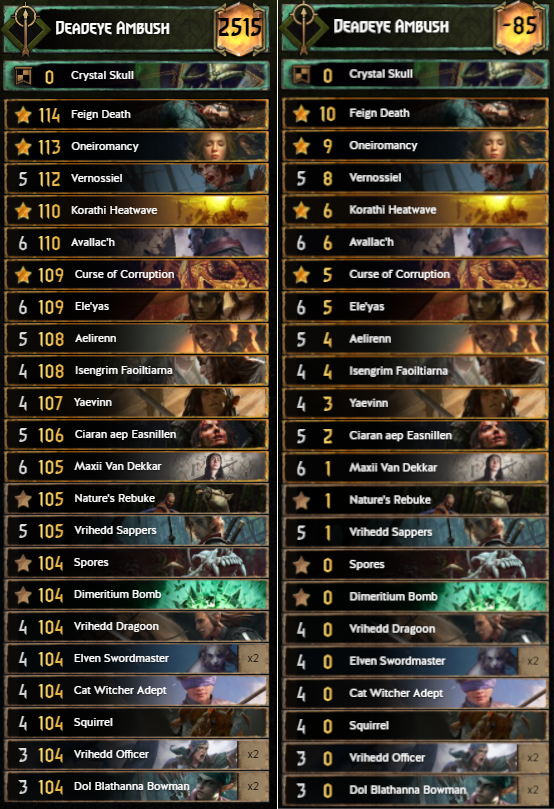
Before starting, let me just introduce some simple syntax to avoid confusion. When I say something costs Xp_N I mean it costs X provisions, at the N base, meaning the number where provision costs start. The two I’ll be using for the rest of this article are Xp₀ and Xp₄. When I say 5p₄ I’m talking about a 5 provision card in the standard, 4 base. And when I say 1p₀ I’m talking about a card that costs 1 in 0 base. Converting between the two is easy, a 4p₄ is equivalent to 0p₀. A 5p₄ is a 1p₀ and so on.

It is much more useful to think about cards from a base of 0 provisions, rather than the standard base of 4. The most obvious and immediately useful reason for this is easily seeing what removing a card will let you add. Cutting a 12p₄ card lets you add 2 8p₄ cards to your deck by upgrading a filler 4p₄ card. There's the extra step there to add 4 for each additional card you're adding to the deck. If you're upgrading a 12p card into 3 cheaper cards, you have 12+4+4, 20 free provisions, so you can add 2 7s and a 6. If you change your mind and decide you want to add 4 cards, now you have 24 free provisions instead of 20, and can add 4 6s. It isn't difficult math, but it's much easier in base 0. Looking at provisions in base 0, it's immediately obvious that by cutting your 8p₀ card, you can add 2 4p₀ cards to the deck, or any other combination of cards that adds up to 8. The extra constant obscures what is really just simple addition when simplified.

Looking at provisions from base 0 also gives you a much better idea of the scale between costs. Functionally, a 6 provision card costs twice as much as a 5 provision. You can play 2 5p₄ cards in your deck for every 6p₄ card you cut by cutting one of your filler cards. 4p₄ cards are "free" since you can't play anything cheaper to fill your deck to the legal limit, so you start paying for your 5 provision cards. The difference between 1 and 2 is a 100% difference, but the identical difference between 5 and 6 is misleadingly small.

Base 0 also has some higher level concepts built-in. Evaluating multi-card combos is something that Gwent novices get wrong constantly. Hunting Pack isn't an 8 for 10 that thins 1, Archespore certainly doesn't cost 8, and Blightmaker combo doesn't cost 9. The correct way to evaluate these is the card you're playing, then how much more you're paying for the other combo piece than you would a filler, as the card coming out of your deck can never be cheaper than that. So in practice, it's much more useful to think about Hunting Pack as an 8 for 6 that thins 1.

In base 0, you get this intuition for free. There's no mismatch in evaluation between cards that pull points out of your deck and any other card. To use the Hunting Pack example again, you're spending 2p₀ to get 8 points and 1 thinning. The cheapest thing you can play is a 0p₀ card, so instead of the extra step to subtract 4, you subtract 0 which leaves the numbers identical.

The evaluation of each half of an Echo card is the same idea, but in reverse. You get to play each of your Echoes twice, but you're using 2 of your 16 turns to do it, instead of one. Meaning instead of playing a filler card plus your Echo, you get to play your Echo twice. So assuming you get play it twice, and the deck thickening is negligible, how many provisions does each half of Ard Gaeth cost? Obviously just halving it doesn't make sense, we again need to subtract that extraneous constant. Working in base 0 this is simple, you can just halve the cost. Ard Gaeth in totality costs 4p₀, and each half of Ard Gaeth costs 2p₀. In base 4, you basically need to convert to base 0, then convert back to base 4 to get to the answer 6p₄.

Provisions starting at 4 was a decision made in early Homecoming development when points were much more linearly tied to their provision value. With these numbers being more and more decoupled, provisions being in base 4 is completely arbitrary and obscures what it actually costs to include cards in your deck. It is much more useful to think about cards in base 0. The math is more intuitive, and insights don't get obscured by the extra constant added into the provision system.